Make A Fractal

Teaching math using art and creativity is fun!  Students learn How To Make A Fractal on this page and if they're like most young people, they'll get a kick out of it while learning about the math of fractals.

What is a Fractal:  In simple terms, it's a never ending pattern.  It's an irregular geometric shape that can be repeatedly subdivided into parts, each of which is a smaller copy of the whole.

Fractal Examples in Nature: clouds, mountainous landscapes, and coastlines.

Koch Snowflake

make a fractal

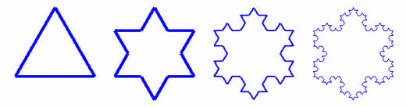
The first fractal we're going to make is called the Koch Snowflake.  It can be constructed from an equilateral triangle and is simple enough to create even for elementary students.  But middle school and even high school students will enjoy because it can be as simple or as complex as the student wants it to be.

Here we go.


Step 1

First draw an equilateral triangle in the middle of the paper.  Draw it large as possible but leave at least an inch of blank space on the sides of the triangle.

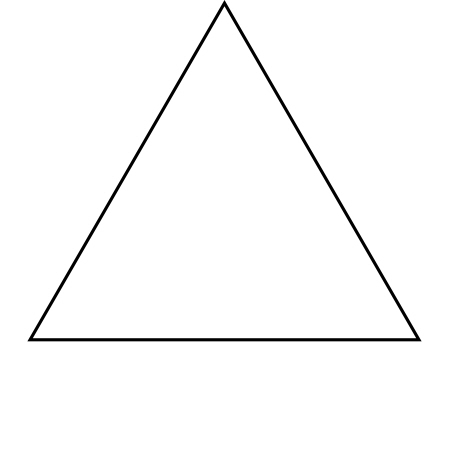Step 2

Using a ruler, divide one of the three sides into three equal pieces.  One way to do this is to measure the length of a side with the ruler.  Then divide your measurement by 3.   Mark off the distance with a pencil starting at the edge of one side of the triangle.  Then mark off the same distance from the point that was just marked. 

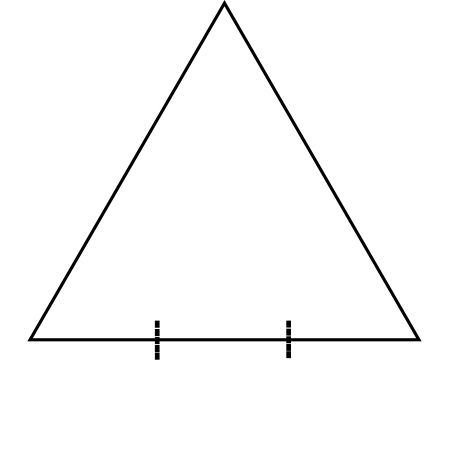Step 3

Next, erase the middle section of the side that was divided into three equal pieces.  See the picture at the right.  

Step 4

Now we are going to draw a line that is the same size as the line we erased from each open end.

The two pieces will meet in the middle at a single point.

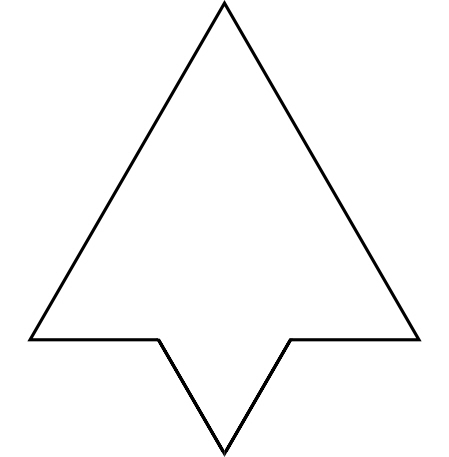Step 5

We will now repeat the same procedure for the other two sides of the triangle.

So after completing all three sides our triangle looks like the picture at he right.  We just completed one iteration.  This is a fancy word mathematicians use for going through a complete process we just did.

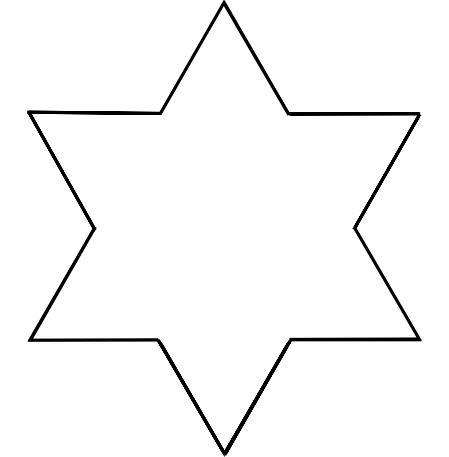Step 6

We will now repeat the same process as above.  The star in the picture above has 12 sides.  So we will repeat 12 times for each of the sides. 

The picture on the right shows the process done to one side.

Now check out the picture on the right... as you can see, we just repeated the same process for all 12 of the sides of our original star.

Now you can do the same thing all over for each side of this new star too.

Now we've just completed two iterations.

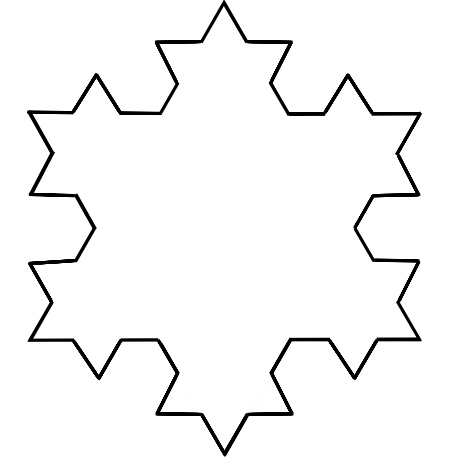This is just one type of fractal.  There are many more and they can even be a lot more complex.  For instance, we could keep repeating the process.  A challenge might be to see how many iterations students can do.

Go to Math Project Ideas page

Go to Learn With Math Games Home

Please note that all fields followed by an asterisk must be filled in.

Please enter the word that you see below.

  

Enjoy this page? Please pay it forward. Here's how...

Would you prefer to share this page with others by linking to it?

  1. Click on the HTML link code below.
  2. Copy and paste it, adding a note of your own, into your blog, a Web page, forums, a blog comment, your Facebook account, or anywhere that someone would find this page valuable.